When Fermat's problem runs into geometric correspondence from below.md

Platonism hits mathematics of The Greatest Math Joke

A quick introduction to the idea known as the Greek proof

Greeks solved $a x^2+b x + c = 0$ semi-geometrically

NOTE: For a complete understanding, please look a little longer, at least for cubic polynomials

What's we proof, known as the Fermat's equation:

$${a, b, c, n} \in \mathbb{N}$$

$$n \geq 3$$

$$a^n + b^n = c^n$$

That's can be rewritten in form:

$${a, b, c, n} \in \mathbb{N}$$

$$n \geq 1$$

$$a^2(a^n) + b^2(b^n) = c^2(c^n)$$

Let's introduce definitions

$$m = a^n$$

$$k = b^n - a^n$$

$$p = c^n - b^n$$

Then:

$$a^2 (m) + b^2 (m + k) = c^2 (m + k + p)$$

Consider this as a question of the existence of a cube, the:

$a' = m$, where any one of a

$b' = m + k$, where any one of b

$c' = m + k + p$, where any one of c

Then:

$$a^2 a' + b^2 b' = c^2 c'$$

$$a' = m$$

$$b' = b + k$$

$$c' = m + k + p$$

Next

$$a' = a^n$$

$$b' = b^n - a^n$$

$$c' = c^n - b^n$$

For any n $a'\neq a, b'\neq b, c'\neq c$.

Then, the cube does not exist for any n > 0. Q.E.D

Objection: You solved the "weaker" version of the problem, the $a^n+b^n = c^n$ is stronger

This statement is related to violation, analytical reasoning in the commission of algebraic transformations.

Simply put, this is your impression, there is a wonderful way to test the strength of the equation that I solved. Do the operations in reverse order and get the same equation (Substitute the variables we defined into the expression).

The question of the existence of a cube is equivalent to the question of the existence of natural solutions.

Objection: How do we know that the “remaining volumes B” for some n will not give an integer amount of “volumes C”

This statement is associated with a violation of the interpretation of the algebraic consequences of geometric laws. Simply put, insufficient geometric intuition. This statement is actually equivalent to the fact that for an n-dimensional cube the Pythagorean thesis does not hold for any faces or volume as a whole.

Fortunately, basic reasoning is enough to show the fallacy of this statement.

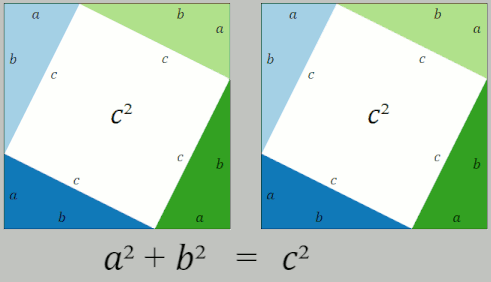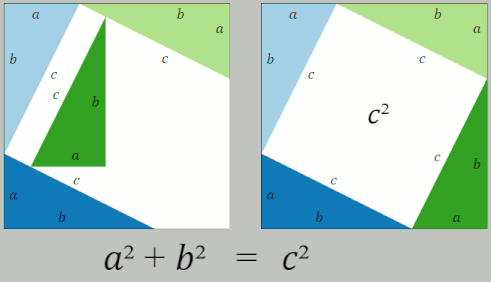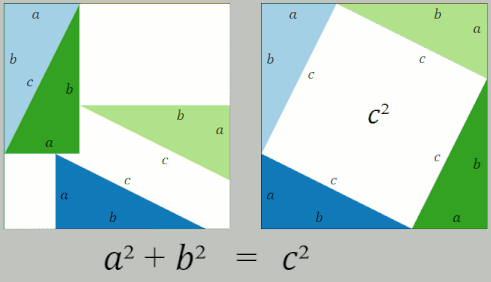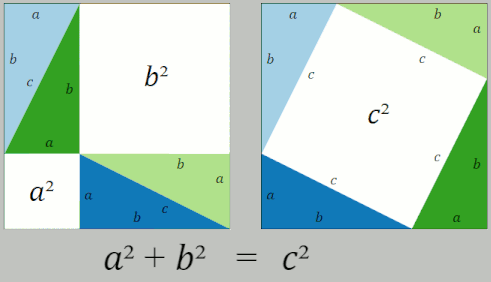
Let's move to two-dimensional space and carry out our reasoning on a plane.

Let's let's look at our equation:

$$a^2 a^n + b^2 b^n = c^2 c^n$$

Then, we can consider $a^n, b^n, c^n$ as AMOUNT of squares (GEOMETRIC FIGURE). YEP, SQUARES (ON SURFACE)!

NOTE: Here and next, when I say heap, I mean multisets of squares of the same area.

Amount of squares in heap A

$$m = a^n$$

The difference is the amount of squares in heap A and B

$$k = b^n - a^n$$

The difference is the amount of squares in heap С and B

$$p = c^n - b^n$$

From above:

Amount of squares in heap B

$$m + k$$

Amount of squares in heap C

$$m + k + p$$

Our equation will again take the form:

$$a^2*(m) + b^2*(m + k) = c^2*(m + k + p)$$

For any squares in heap A there is a square from heap B whose sum will give a square from heap C.

The question then becomes whether. Is some remain amount of squares B that's will create an integer amount of squares C.

Let's write it as system of equation. Where first represent m sums of all A + B = C. And second remain amount of squares from heap B that's will create an integer amount of squares of heap C

$$\begin{cases} (a^2 + b^2) m = c^2 m\\\ b^2 \cdot k = c^2 \cdot (k + p) \end{cases}$$

Next:

$$\begin{cases} a^2 + b^2 = c^2\\\ b^2 \cdot k = c^2 \cdot (k + p) \end{cases}$$

Because, ${a, b, c} \in \mathbb{N}$, Pythagorean thesis:

$a^2 + b^2 = c^2$ work for m number of squares in heap A, B, C, and this squares alwayes exist, but when, and only when if $a < b < c$.

$b^2*k = c^2*(k+p)$

Next

$a^2 + b^2 = c^2$

$b^2*k = c^2*(k+p)$

Since $a < b < c$. Hence $b^2 < c^2$. Then if valid only if assume $k > k + p$.

$p < 0$

Let's substitute p

$c^n - b^n < 0$

$c^n < b^n$

That's contradiction. Q.E.D.

It is not difficult to understand that one can reason in the same way about n-dimensional cubes. Not as about their representations in space, but as about their AMOUNT.

In other words, the Pythagorean thesis for n-dimensions also known as Fermat's Theorem:

$a^n + b^n = c^n$

implies the ratio of the volume of n-dimensional cubes. And it cannot be violated either with an increase in “AMOUNT” or another word's with an increase in dimension.

Foundations of algebra and geometric comparability

I get questions like why Pythagoras’ thesis is true for the faces of a cube... Oh God... Because algebra has its origins in Euclid's geometry. How do we know that 4 · π · R is the area of a sphere? Okay, how do we know that x^2 is the area of a square?

Now hold on to your pants tighter. How do we even know how to solve polynomials? Did the magic fairy give us the discriminant formula? Secret, watch the video at the beginning, we can solve polynomials of at least the second degree, because we use the Pythagorean thesis. If we deny the Pythagorean thesis, we generally do not know how to solve polynomials, and we should not even take on Fermat’s Theorem, because all algebra collapses into a singularity point.

Now to the question of the Pythagorean thesis.

There was this guy named Kurt Gödel, and he said this thing: any formal system is either incomplete or contradictory. It is quite obvious that for algebra this will be the Pythagorean thesis, since it lies outside the boundaries of algebra. And we simply believe that this is so, whereas for geometry it will be the Pythagorean theorem, which is precisely proven by the methods of planimetry. For algebra, the formula a^2 + b^2 = c^2, which establishes the ratio of areas, sides, the existence of trigonometric functions, etc. this will be the thesis. Attempts to prove the thesis and its consequences do not stand up to criticism.

Now, I will once again ask you to watch the video at the beginning. As it is not difficult to understand, all the founders of algebra were excellent geometers.

Ask yourself why Fermat’s equation has such strange conditions, and why it “accidentally” turned out to be nearby with Pythagorean thesis. Farm couldn't solve it? After he brought it out himself? Do you really think Fermat is an idiot after spending 350 years looking for a cube with a face such that a^2 + b^2 != c^2?

The funny thing about this whole story is that I am a Platonist, but I am looking for the manifestations of numbers, and not the numbers themselves, how crazy do you have to be to get lost in the palaces of your own mind and lose all connection with reality.